[足式机器人]Part4 南科大高等机器人控制课 Ch09 Dynamics of Open Chains
本文仅供学习使用
本文参考:
B站:CLEAR_LAB
笔者带更新-运动学
课程主讲教师:
Prof. Wei Zhang
南科大高等机器人控制课 Ch09 Dynamics of Open Chains
- 1. Introduction
- 1.1 From Single Rigid Body to Open Chains
- 1.2 Preview of Open-Chain Dynamics
- 1.3 Lagrangian VS. Newton-Euler Methods
- 2. Inverse Dynamics: Recursive Newton-Euler Algorithm(RNEA)
- 2.1 RNEA: Notations
- 2.1.1 RNEA: Velocity and Accel. Propagation(Forward Pass)
- 2.1.2 RNEA: Force Propagation(Backward Pass)
- 2.1.3 Recursive Newton-Euler Algorithm
- 3. Analytical Form of the Dynamics Model
- 3.1 Structures in Dynamic Equation
- 3.2 Properties of Dynamics Model of Multi-Body Systems
- 4. Forward Dynamics Algorithms
- 4.1 Forward Dynamics Problem
- 4.2 Caculations of h and M
- 4.3 Forward Dynamics Algorithms
- 4.4 More Discussions
1. Introduction
1.1 From Single Rigid Body to Open Chains
- Recall Newton-Euler Equation for a single rigid body:
F = d d t H = I A + V ~ ∗ I V \mathcal{F} =\frac{\mathrm{d}}{\mathrm{d}t}\mathcal{H} =\mathcal{I} \mathcal{A} +\tilde{\mathcal{V}}^*\mathcal{I} \mathcal{V} F=dtdH=IA+V~∗IV - Open chains consists of multiple rigid links connected through joints
- Dynamics of adjacent links are coupled
- This lecture: model multi-body dynamics subject to joint constrainsts.
1.2 Preview of Open-Chain Dynamics
-
Equations of Motion are a set of 2nd-order differential equations:
τ = M ( θ ) θ ¨ + c ( θ , θ ˙ ) \tau =M\left( \theta \right) \ddot{\theta}+c\left( \theta ,\dot{\theta} \right) τ=M(θ)θ¨+c(θ,θ˙)
θ ∈ R n \theta \in \mathbb{R} ^n θ∈Rn : vector of joint variables ;
τ ∈ R n \tau \in \mathbb{R} ^n τ∈Rn : vector of joint forces/torques(与之前的符号不一致);
M ( θ ) ∈ R n × n M\left( \theta \right) \in \mathbb{R} ^{n\times n} M(θ)∈Rn×n : mass matrix
c ( θ , θ ˙ ) ∈ R n c\left( \theta ,\dot{\theta} \right) \in \mathbb{R} ^n c(θ,θ˙)∈Rn : forces that lump together centripetal, Coriolis, gravity, friction terms, and torques induced by external forces. These terms depends on θ \theta θ and θ ˙ \dot{\theta} θ˙ -
Forward dynamics : Determine accecleration θ ¨ \ddot{\theta} θ¨ given the state ( θ , θ ˙ ) \left( \theta ,\dot{\theta} \right) (θ,θ˙) and the joint forces/torques
θ ¨ ← F D ( τ , θ , θ ˙ , F e x t ) \ddot{\theta}\gets FD\left( \tau ,\theta ,\dot{\theta},\mathcal{F} _{\mathrm{ext}} \right) θ¨←FD(τ,θ,θ˙,Fext) -
Inverse dynamics : Finding torques/forces given state ( θ , θ ˙ ) \left( \theta ,\dot{\theta} \right) (θ,θ˙) and desired acceleration θ ¨ \ddot{\theta} θ¨ (Given desired motion, find the required torque to generate the desired motion)
τ ← I D ( θ , θ ˙ , θ ¨ , F e x t ) \tau \gets ID\left( \theta ,\dot{\theta},\ddot{\theta},\mathcal{F} _{\mathrm{ext}} \right) τ←ID(θ,θ˙,θ¨,Fext)
1.3 Lagrangian VS. Newton-Euler Methods
- There are typically two ways to derive the equation of motion for an open-chain robot: Lagrangian method and Newton-Euler method
Lagrangian Formulation : Energy-based method ; Dynamic equations in closed form ; Often used for study of dynamic properties and analysis of control methods
Newton-Euler Formulation : Balance of forces/torques ; Dynamic equations in numeric/recuisive form ; Often used for numerical solution of forward/inverse dynamics
We focus on Newton-Euler Formulation
2. Inverse Dynamics: Recursive Newton-Euler Algorithm(RNEA)
2.1 RNEA: Notations

-
Number bodies : 1 to N N N
Parents : p ( i ) : p ( 3 ) = { 2 } , p ( 4 ) = { 2 } p\left( i \right) :p\left( 3 \right) =\left\{ 2 \right\} ,p\left( 4 \right) =\left\{ 2 \right\} p(i):p(3)={2},p(4)={2}
Children : c ( i ) : c ( 2 ) = { 3 , 4 } , c ( 1 ) = { 2 } c\left( i \right) :c\left( 2 \right) =\left\{ 3,4 \right\} ,c\left( 1 \right) =\left\{ 2 \right\} c(i):c(2)={3,4},c(1)={2} -
Joint i i i connects p ( i ) p\left( i \right) p(i) to i i i
-
Frame { i } \left\{ i \right\} {i} attached to body i i i at the joint
-
S i \mathcal{S} _{\mathrm{i}} Si : Spatial velocity (screw axis) of joint i i i
-
V i \mathcal{V} _{\mathrm{i}} Vi and A i \mathcal{A} _{\mathrm{i}} Ai : spatial velocity and acceleration of body i i i
-
F i \mathcal{F} _{\mathrm{i}} Fi : force(wrench) onto body i i i from body p ( i ) p\left( i \right) p(i)
-
Note : By default, all vectors ( S i , V i , F i ) \left( \mathcal{S} _{\mathrm{i}},\mathcal{V} _{\mathrm{i}},\mathcal{F} _{\mathrm{i}} \right) (Si,Vi,Fi) are expressed in local frame { i } \left\{ i \right\} {i}
2.1.1 RNEA: Velocity and Accel. Propagation(Forward Pass)
Goal: Given joint velocity θ ˙ \dot{\theta} θ˙ and acceleration θ ¨ \ddot{\theta} θ¨ , compute the body spatial velocity V i \mathcal{V} _{\mathrm{i}} Vi and spatial acceleration A i \mathcal{A} _{\mathrm{i}} Ai
- Velocity Propagation : V i i = [ X p ( i ) i ] V p ( i ) p ( i ) + S i i θ ˙ i \mathcal{V} _{\mathrm{i}}^{i}=\left[ X_{\mathrm{p}\left( i \right)}^{i} \right] \mathcal{V} _{\mathrm{p}\left( i \right)}^{p\left( i \right)}+\mathcal{S} _{\mathrm{i}}^{i}\dot{\theta}_{\mathrm{i}} Vii=[Xp(i)i]Vp(i)p(i)+Siiθ˙i
- Accel Propagation : A i i = [ X p ( i ) i ] A p ( i ) p ( i ) + V ~ i i S i i θ ˙ i + S i i θ ¨ i \mathcal{A} _{\mathrm{i}}^{i}=\left[ X_{\mathrm{p}\left( i \right)}^{i} \right] \mathcal{A} _{\mathrm{p}\left( i \right)}^{p\left( i \right)}+\tilde{\mathcal{V}}_{\mathrm{i}}^{i}\mathcal{S} _{\mathrm{i}}^{i}\dot{\theta}_{\mathrm{i}}+\mathcal{S} _{\mathrm{i}}^{i}\ddot{\theta}_{\mathrm{i}} Aii=[Xp(i)i]Ap(i)p(i)+V~iiSiiθ˙i+Siiθ¨i
从机架侧开始计算
2.1.2 RNEA: Force Propagation(Backward Pass)
Goal : Given body spatial velocity V i \mathcal{V} _{\mathrm{i}} Vi amd spatial acceleration A i \mathcal{A} _{\mathrm{i}} Ai, compute the joint wrench F i \mathcal{F} _{\mathrm{i}} Fi and the corresponding torque τ i = S i T F i \tau _{\mathrm{i}}={\mathcal{S} _{\mathrm{i}}}^{\mathrm{T}}\mathcal{F} _{\mathrm{i}} τi=SiTFi
{ F i = I i A i + V ~ i ∗ I i V i + ∑ j ∈ c ( i ) F j τ i = S i T F i \begin{cases} \mathcal{F} _{\mathrm{i}}=\mathcal{I} _{\mathrm{i}}\mathcal{A} _{\mathrm{i}}+{\tilde{\mathcal{V}}_{\mathrm{i}}}^*\mathcal{I} _{\mathrm{i}}\mathcal{V} _{\mathrm{i}}+\sum\nolimits_{j\in c\left( i \right)}^{}{\mathcal{F} _{\mathrm{j}}}\\ \tau _{\mathrm{i}}={\mathcal{S} _{\mathrm{i}}}^{\mathrm{T}}\mathcal{F} _{\mathrm{i}}\\ \end{cases} {Fi=IiAi+V~i∗IiVi+∑j∈c(i)Fjτi=SiTFi
从末端执行构件处开始计算:
- Body 4:
F 4 + F G 4 = I 4 A 4 + V ~ 4 ∗ I 4 V 4 ⇒ F 4 = I 4 A 4 + V ~ 4 ∗ I 4 V 4 − F G 4 , F G 4 = I 4 A G 4 = I 4 [ X O 4 ] A G O \mathcal{F} _4+\mathcal{F} _{\mathrm{G}4}=\mathcal{I} _4\mathcal{A} _4+{\tilde{\mathcal{V}}_4}^*\mathcal{I} _4\mathcal{V} _4 \\ \Rightarrow \mathcal{F} _4=\mathcal{I} _4\mathcal{A} _4+{\tilde{\mathcal{V}}_4}^*\mathcal{I} _4\mathcal{V} _4-\mathcal{F} _{\mathrm{G}4},\mathcal{F} _{\mathrm{G}4}=\mathcal{I} _4\mathcal{A} _{\mathrm{G}}^{4}=\mathcal{I} _4\left[ X_{\mathrm{O}}^{4} \right] \mathcal{A} _{\mathrm{G}}^{O} F4+FG4=I4A4+V~4∗I4V4⇒F4=I4A4+V~4∗I4V4−FG4,FG4=I4AG4=I4[XO4]AGO
τ 4 = S 4 T F 4 \tau _4={\mathcal{S} _4}^{\mathrm{T}}\mathcal{F} _4 τ4=S4TF4 - Body 2:
F 2 = I 2 A 2 + V ~ 2 ∗ I 2 V 2 + F 4 + F 3 − F G 2 , F G 2 = I 2 [ X O 2 ] A G 2 O τ 2 = S 2 T F 2 \mathcal{F} _2=\mathcal{I} _2\mathcal{A} _2+{\tilde{\mathcal{V}}_2}^*\mathcal{I} _2\mathcal{V} _2+\mathcal{F} _4+\mathcal{F} _3-\mathcal{F} _{\mathrm{G}2},\mathcal{F} _{\mathrm{G}2}=\mathcal{I} _2\left[ X_{\mathrm{O}}^{2} \right] \mathcal{A} _{\mathrm{G}2}^{O} \\ \tau _2={\mathcal{S} _2}^{\mathrm{T}}\mathcal{F} _2 F2=I2A2+V~2∗I2V2+F4+F3−FG2,FG2=I2[XO2]AG2Oτ2=S2TF2
2.1.3 Recursive Newton-Euler Algorithm
τ ← R N E A ( θ , θ ˙ , θ ¨ , F e x t ; M o d e l ) \tau \gets RNEA\left( \theta ,\dot{\theta},\ddot{\theta},\mathcal{F} _{\mathrm{ext}};Model \right) τ←RNEA(θ,θ˙,θ¨,Fext;Model)
Initialize : V 0 = 0 , A 0 = − A G \mathcal{V} _0=0,\mathcal{A} _0=-\mathcal{A} _{\mathrm{G}} V0=0,A0=−AG (without gravity “trick” modify F i = I i A i + V ~ i ∗ I i V i − I i [ X O i ] A G i O \mathcal{F} _{\mathrm{i}}=\mathcal{I} _{\mathrm{i}}\mathcal{A} _{\mathrm{i}}+{\tilde{\mathcal{V}}_{\mathrm{i}}}^*\mathcal{I} _{\mathrm{i}}\mathcal{V} _{\mathrm{i}}-\mathcal{I} _{\mathrm{i}}\left[ X_{\mathrm{O}}^{i} \right] \mathcal{A} _{\mathrm{Gi}}^{O} Fi=IiAi+V~i∗IiVi−Ii[XOi]AGiO)
- Forward pass : For i = 1 i=1 i=1 to N N N
V i i = [ X p ( i ) i ] V p ( i ) p ( i ) + S i i θ ˙ i \mathcal{V} _{\mathrm{i}}^{i}=\left[ X_{\mathrm{p}\left( i \right)}^{i} \right] \mathcal{V} _{\mathrm{p}\left( i \right)}^{p\left( i \right)}+\mathcal{S} _{\mathrm{i}}^{i}\dot{\theta}_{\mathrm{i}} Vii=[Xp(i)i]Vp(i)p(i)+Siiθ˙i
A i i = [ X p ( i ) i ] A p ( i ) p ( i ) + V ~ i i S i i θ ˙ i + S i i θ ¨ i \mathcal{A} _{\mathrm{i}}^{i}=\left[ X_{\mathrm{p}\left( i \right)}^{i} \right] \mathcal{A} _{\mathrm{p}\left( i \right)}^{p\left( i \right)}+\tilde{\mathcal{V}}_{\mathrm{i}}^{i}\mathcal{S} _{\mathrm{i}}^{i}\dot{\theta}_{\mathrm{i}}+\mathcal{S} _{\mathrm{i}}^{i}\ddot{\theta}_{\mathrm{i}} Aii=[Xp(i)i]Ap(i)p(i)+V~iiSiiθ˙i+Siiθ¨i
F i = I i A i + V ~ i ∗ I i V i \mathcal{F} _{\mathrm{i}}=\mathcal{I} _{\mathrm{i}}\mathcal{A} _{\mathrm{i}}+{\tilde{\mathcal{V}}_{\mathrm{i}}}^*\mathcal{I} _{\mathrm{i}}\mathcal{V} _{\mathrm{i}} Fi=IiAi+V~i∗IiVi - Backward pass : For i = N i=N i=N to 1
τ i = S i T F i \tau _{\mathrm{i}}={\mathcal{S} _{\mathrm{i}}}^{\mathrm{T}}\mathcal{F} _{\mathrm{i}} τi=SiTFi
F p ( i ) = F p ( i ) + [ X i p ( i ) ] F i \mathcal{F} _{\mathrm{p}\left( i \right)}=\mathcal{F} _{\mathrm{p}\left( i \right)}+\left[ X_{\mathrm{i}}^{p\left( i \right)} \right] \mathcal{F} _{\mathrm{i}} Fp(i)=Fp(i)+[Xip(i)]Fi
3. Analytical Form of the Dynamics Model
3.1 Structures in Dynamic Equation
Jacobian of each link(body) : J 1 , ⋯ , J 4 J_1,\cdots ,J_4 J1,⋯,J4
J i J_{\mathrm{i}} Ji : denote the Jacobian of body(Link) i i i , i.e. V i = J i θ ˙ = [ J i 1 J i 2 J i 3 J i 4 ] [ θ ˙ 1 θ ˙ 2 θ ˙ 3 θ ˙ 4 ] \mathcal{V} _{\mathrm{i}}=J_{\mathrm{i}}\dot{\theta}=\left[ \begin{matrix} J_{\mathrm{i}1}& J_{\mathrm{i}2}& J_{\mathrm{i}3}& J_{\mathrm{i}4}\\ \end{matrix} \right] \left[ \begin{array}{c} \dot{\theta}_1\\ \dot{\theta}_2\\ \dot{\theta}_3\\ \dot{\theta}_4\\ \end{array} \right] Vi=Jiθ˙=[Ji1Ji2Ji3Ji4] θ˙1θ˙2θ˙3θ˙4
e.g. V 1 = J 1 θ ˙ = [ δ 11 S 1 δ 12 S 2 δ 13 S 3 δ 14 S 4 ] [ θ ˙ 1 θ ˙ 2 θ ˙ 3 θ ˙ 4 ] = [ S 1 0 0 0 ] [ θ ˙ 1 θ ˙ 2 θ ˙ 3 θ ˙ 4 ] δ i j = { 1 , i f j o i n t j s u p p o r t b o d y i 0 , o t h e r w i s e \mathcal{V} _1=J_1\dot{\theta}=\left[ \begin{matrix} \delta _{11}\mathcal{S} _1& \delta _{12}\mathcal{S} _2& \delta _{13}\mathcal{S} _3& \delta _{14}\mathcal{S} _4\\ \end{matrix} \right] \left[ \begin{array}{c} \dot{\theta}_1\\ \dot{\theta}_2\\ \dot{\theta}_3\\ \dot{\theta}_4\\ \end{array} \right] =\left[ \begin{matrix} \mathcal{S} _1& 0& 0& 0\\ \end{matrix} \right] \left[ \begin{array}{c} \dot{\theta}_1\\ \dot{\theta}_2\\ \dot{\theta}_3\\ \dot{\theta}_4\\ \end{array} \right] \\ \delta _{\mathrm{ij}}=\begin{cases} 1, if\,\,joint\,\,\mathrm{j} support\,\,body\,\,\mathrm{i}\\ 0, otherwise\\ \end{cases} V1=J1θ˙=[δ11S1δ12S2δ13S3δ14S4] θ˙1θ˙2θ˙3θ˙4 =[S1000] θ˙1θ˙2θ˙3θ˙4 δij={1,ifjointjsupportbodyi0,otherwise
V 2 = J 2 θ ˙ = [ S 1 S 2 0 0 ] θ ˙ ⇒ V 2 2 = [ [ X 1 2 ] S 2 1 S 2 2 0 0 ] θ ˙ \mathcal{V} _2=J_2\dot{\theta}=\left[ \begin{matrix} \mathcal{S} _1& \mathcal{S} _2& 0& 0\\ \end{matrix} \right] \dot{\theta}\Rightarrow \mathcal{V} _{2}^{2}=\left[ \left[ X_{1}^{2} \right] \begin{matrix} \mathcal{S} _{2}^{1}& \mathcal{S} _{2}^{2}& 0& 0\\ \end{matrix} \right] \dot{\theta} V2=J2θ˙=[S1S200]θ˙⇒V22=[[X12]S21S2200]θ˙
see the two-body example:
- Forward pass :
V 1 = S 1 θ ˙ 1 \mathcal{V} _1=\mathcal{S} _1\dot{\theta}_1 V1=S1θ˙1 , V 2 2 = [ [ X 1 2 ] S 2 1 S 2 2 ] [ θ ˙ 1 θ ˙ 2 ] \mathcal{V} _{2}^{2}=\left[ \begin{matrix} \left[ X_{1}^{2} \right] \mathcal{S} _{2}^{1}& \mathcal{S} _{2}^{2}\\ \end{matrix} \right] \left[ \begin{array}{c} \dot{\theta}_1\\ \dot{\theta}_2\\ \end{array} \right] V22=[[X12]S21S22][θ˙1θ˙2]
A 1 , A 2 \mathcal{A} _1, \mathcal{A} _2 A1,A2 - Backward pass :
F 2 = I 2 A 2 + V ~ 2 ∗ I 2 V 2 − F 2 e x t − F 2 G F 1 = I 1 A 1 + V ~ 1 ∗ I 1 V 1 − F 1 e x t − F 1 G + [ X 2 1 ] ( I 2 A 2 + V ~ 2 ∗ I 2 V 2 − F 2 e x t − F 2 G ) τ 2 = S 2 T F 2 τ 1 = S 1 T F 1 \mathcal{F} _2=\mathcal{I} _2\mathcal{A} _2+{\tilde{\mathcal{V}}_2}^*\mathcal{I} _2\mathcal{V} _2-\mathcal{F} _{2\mathrm{ext}}-\mathcal{F} _{2\mathrm{G}} \\ \mathcal{F} _1=\mathcal{I} _1\mathcal{A} _1+{\tilde{\mathcal{V}}_1}^*\mathcal{I} _1\mathcal{V} _1-\mathcal{F} _{1\mathrm{ext}}-\mathcal{F} _{1\mathrm{G}}+\left[ X_{2}^{1} \right] \left( \mathcal{I} _2\mathcal{A} _2+{\tilde{\mathcal{V}}_2}^*\mathcal{I} _2\mathcal{V} _2-\mathcal{F} _{2\mathrm{ext}}-\mathcal{F} _{2\mathrm{G}} \right) \\ \tau _2={\mathcal{S} _2}^{\mathrm{T}}\mathcal{F} _2 \\ \tau _1={\mathcal{S} _1}^{\mathrm{T}}\mathcal{F} _1 F2=I2A2+V~2∗I2V2−F2ext−F2GF1=I1A1+V~1∗I1V1−F1ext−F1G+[X21](I2A2+V~2∗I2V2−F2ext−F2G)τ2=S2TF2τ1=S1TF1
Overall torque expression :
τ 1 = S 1 T F 1 = S 1 T ( I 1 A 1 + V ~ 1 ∗ I 1 V 1 − F 1 e x t − F 1 G + [ X 2 1 ] ( I 2 A 2 + V ~ 2 ∗ I 2 V 2 − F 2 e x t − F 2 G ) ) = S 1 T ( I 1 A 1 + V ~ 1 ∗ I 1 V 1 ) + ( [ X 1 2 ] S 1 ) T ( I 2 A 2 + V ~ 2 ∗ I 2 V 2 ) − S 1 T ( F 1 e x t + F 1 G + [ X 2 1 ] ( F 2 e x t + F 2 G ) ) \tau _1={\mathcal{S} _1}^{\mathrm{T}}\mathcal{F} _1={\mathcal{S} _1}^{\mathrm{T}}\left( \mathcal{I} _1\mathcal{A} _1+{\tilde{\mathcal{V}}_1}^*\mathcal{I} _1\mathcal{V} _1-\mathcal{F} _{1\mathrm{ext}}-\mathcal{F} _{1\mathrm{G}}+\left[ X_{2}^{1} \right] \left( \mathcal{I} _2\mathcal{A} _2+{\tilde{\mathcal{V}}_2}^*\mathcal{I} _2\mathcal{V} _2-\mathcal{F} _{2\mathrm{ext}}-\mathcal{F} _{2\mathrm{G}} \right) \right) \\ ={\mathcal{S} _1}^{\mathrm{T}}\left( \mathcal{I} _1\mathcal{A} _1+{\tilde{\mathcal{V}}_1}^*\mathcal{I} _1\mathcal{V} _1 \right) +\left( \left[ X_{1}^{2} \right] \mathcal{S} _1 \right) ^{\mathrm{T}}\left( \mathcal{I} _2\mathcal{A} _2+{\tilde{\mathcal{V}}_2}^*\mathcal{I} _2\mathcal{V} _2 \right) -{\mathcal{S} _1}^{\mathrm{T}}\left( \mathcal{F} _{1\mathrm{ext}}+\mathcal{F} _{1\mathrm{G}}+\left[ X_{2}^{1} \right] \left( \mathcal{F} _{2\mathrm{ext}}+\mathcal{F} _{2\mathrm{G}} \right) \right) τ1=S1TF1=S1T(I1A1+V~1∗I1V1−F1ext−F1G+[X21](I2A2+V~2∗I2V2−F2ext−F2G))=S1T(I1A1+V~1∗I1V1)+([X12]S1)T(I2A2+V~2∗I2V2)−S1T(F1ext+F1G+[X21](F2ext+F2G))
due to motion of body 1 and 2 and external force of body 1 and 2
τ = [ τ 1 τ 2 ] = [ S 1 T ( I 1 A 1 + V ~ 1 ∗ I 1 V 1 ) + ( [ X 1 2 ] S 1 ) T ( I 2 A 2 + V ~ 2 ∗ I 2 V 2 ) − S 1 T ( F 1 e x t + F 1 G + [ X 2 1 ] ( F 2 e x t + F 2 G ) ) S 2 T ( I 2 A 2 + V ~ 2 ∗ I 2 V 2 − F 2 e x t − F 2 G ) ] = [ S 1 T 0 ] ( I 1 A 1 + V ~ 1 ∗ I 1 V 1 − F 1 e x t − F 1 G ) + [ ( [ X 1 2 ] S 1 ) T S 2 T ] ( I 2 A 2 + V ~ 2 ∗ I 2 V 2 − F 2 e x t − F 2 G ) = J 1 T ( I 1 A 1 + V ~ 1 ∗ I 1 V 1 − F 1 e x t − F 1 G ) + J 2 T ( I 2 A 2 + V ~ 2 ∗ I 2 V 2 − F 2 e x t − F 2 G ) \tau =\left[ \begin{array}{c} \tau _1\\ \tau _2\\ \end{array} \right] =\left[ \begin{array}{c} {\mathcal{S} _1}^{\mathrm{T}}\left( \mathcal{I} _1\mathcal{A} _1+{\tilde{\mathcal{V}}_1}^*\mathcal{I} _1\mathcal{V} _1 \right) +\left( \left[ X_{1}^{2} \right] \mathcal{S} _1 \right) ^{\mathrm{T}}\left( \mathcal{I} _2\mathcal{A} _2+{\tilde{\mathcal{V}}_2}^*\mathcal{I} _2\mathcal{V} _2 \right) -{\mathcal{S} _1}^{\mathrm{T}}\left( \mathcal{F} _{1\mathrm{ext}}+\mathcal{F} _{1\mathrm{G}}+\left[ X_{2}^{1} \right] \left( \mathcal{F} _{2\mathrm{ext}}+\mathcal{F} _{2\mathrm{G}} \right) \right)\\ {\mathcal{S} _2}^{\mathrm{T}}\left( \mathcal{I} _2\mathcal{A} _2+{\tilde{\mathcal{V}}_2}^*\mathcal{I} _2\mathcal{V} _2-\mathcal{F} _{2\mathrm{ext}}-\mathcal{F} _{2\mathrm{G}} \right)\\ \end{array} \right] \\ =\left[ \begin{array}{c} {\mathcal{S} _1}^{\mathrm{T}}\\ 0\\ \end{array} \right] \left( \mathcal{I} _1\mathcal{A} _1+{\tilde{\mathcal{V}}_1}^*\mathcal{I} _1\mathcal{V} _1-\mathcal{F} _{1\mathrm{ext}}-\mathcal{F} _{1\mathrm{G}} \right) +\left[ \begin{array}{c} \left( \left[ X_{1}^{2} \right] \mathcal{S} _1 \right) ^{\mathrm{T}}\\ {\mathcal{S} _2}^{\mathrm{T}}\\ \end{array} \right] \left( \mathcal{I} _2\mathcal{A} _2+{\tilde{\mathcal{V}}_2}^*\mathcal{I} _2\mathcal{V} _2-\mathcal{F} _{2\mathrm{ext}}-\mathcal{F} _{2\mathrm{G}} \right) \\ ={J_1}^{\mathrm{T}}\left( \mathcal{I} _1\mathcal{A} _1+{\tilde{\mathcal{V}}_1}^*\mathcal{I} _1\mathcal{V} _1-\mathcal{F} _{1\mathrm{ext}}-\mathcal{F} _{1\mathrm{G}} \right) +{J_2}^{\mathrm{T}}\left( \mathcal{I} _2\mathcal{A} _2+{\tilde{\mathcal{V}}_2}^*\mathcal{I} _2\mathcal{V} _2-\mathcal{F} _{2\mathrm{ext}}-\mathcal{F} _{2\mathrm{G}} \right) τ=[τ1τ2]= S1T(I1A1+V~1∗I1V1)+([X12]S1)T(I2A2+V~2∗I2V2)−S1T(F1ext+F1G+[X21](F2ext+F2G))S2T(I2A2+V~2∗I2V2−F2ext−F2G) =[S1T0](I1A1+V~1∗I1V1−F1ext−F1G)+[([X12]S1)TS2T](I2A2+V~2∗I2V2−F2ext−F2G)=J1T(I1A1+V~1∗I1V1−F1ext−F1G)+J2T(I2A2+V~2∗I2V2−F2ext−F2G)
Overall : in general with N-links / Joints
τ = ∑ i = 1 N J i T ( I i A i + V ~ i ∗ I i V i − F i e x t − F i G ) \tau =\sum_{i=1}^N{{J_{\mathrm{i}}}^{\mathrm{T}}\left( \mathcal{I} _{\mathrm{i}}\mathcal{A} _{\mathrm{i}}+{\tilde{\mathcal{V}}_{\mathrm{i}}}^*\mathcal{I} _{\mathrm{i}}\mathcal{V} _{\mathrm{i}}-\mathcal{F} _{\mathrm{iext}}-\mathcal{F} _{\mathrm{iG}} \right)} τ=i=1∑NJiT(IiAi+V~i∗IiVi−Fiext−FiG)
V i = J i θ ˙ A i = V ˙ i = ( J ˙ i θ ˙ + J i θ ¨ + V ~ i J i θ ˙ ) \mathcal{V} _{\mathrm{i}}=J_{\mathrm{i}}\dot{\theta} \\ \mathcal{A} _{\mathrm{i}}=\dot{\mathcal{V}}_{\mathrm{i}}=\left( \dot{J}_{\mathrm{i}}\dot{\theta}+J_{\mathrm{i}}\ddot{\theta}+\tilde{\mathcal{V}}_{\mathrm{i}}J_{\mathrm{i}}\dot{\theta} \right) Vi=Jiθ˙Ai=V˙i=(J˙iθ˙+Jiθ¨+V~iJiθ˙)
上式看上去难以理解,尤其是加速度旋量,本质上是因为在构件坐标系下的求导,相当于需要对运动基向量求导所产生的加速度
带入可得:
τ = ∑ i = 1 N J i T I i J i θ ¨ + ∑ i = 1 N J i T ( I i J ˙ i + I i V ~ i J i + V ~ i ∗ I i J i ) θ ˙ \tau =\sum_{i=1}^N{{J_{\mathrm{i}}}^{\mathrm{T}}\mathcal{I} _{\mathrm{i}}J_{\mathrm{i}}}\ddot{\theta}+\sum_{i=1}^N{{J_{\mathrm{i}}}^{\mathrm{T}}\left( \mathcal{I} _{\mathrm{i}}\dot{J}_{\mathrm{i}}+\mathcal{I} _{\mathrm{i}}\tilde{\mathcal{V}}_{\mathrm{i}}J_{\mathrm{i}}+{\tilde{\mathcal{V}}_{\mathrm{i}}}^*\mathcal{I} _{\mathrm{i}}J_{\mathrm{i}} \right)}\dot{\theta} τ=i=1∑NJiTIiJiθ¨+i=1∑NJiT(IiJ˙i+IiV~iJi+V~i∗IiJi)θ˙
∑ i = 1 N J i T I i J i = M ( θ ) , ∑ i = 1 N J i T ( I i J ˙ i + I i V ~ i J i + V ~ i ∗ I i J i ) = c ( θ , θ ˙ ) \sum_{i=1}^N{{J_{\mathrm{i}}}^{\mathrm{T}}\mathcal{I} _{\mathrm{i}}J_{\mathrm{i}}}=M\left( \theta \right) ,\sum_{i=1}^N{{J_{\mathrm{i}}}^{\mathrm{T}}\left( \mathcal{I} _{\mathrm{i}}\dot{J}_{\mathrm{i}}+\mathcal{I} _{\mathrm{i}}\tilde{\mathcal{V}}_{\mathrm{i}}J_{\mathrm{i}}+{\tilde{\mathcal{V}}_{\mathrm{i}}}^*\mathcal{I} _{\mathrm{i}}J_{\mathrm{i}} \right)}=c\left( \theta ,\dot{\theta} \right) ∑i=1NJiTIiJi=M(θ),∑i=1NJiT(IiJ˙i+IiV~iJi+V~i∗IiJi)=c(θ,θ˙) , τ G = ∑ i = 1 N J i T I i [ X O i ] ( − A i G O ) \tau _G=\sum_{i=1}^N{{J_{\mathrm{i}}}^{\mathrm{T}}\mathcal{I} _{\mathrm{i}}\left[ X_{\mathrm{O}}^{i} \right]}\left( -\mathcal{A} _{\mathrm{iG}}^{O} \right) τG=∑i=1NJiTIi[XOi](−AiGO)
最终理解: τ = M ( θ ) θ ¨ + c ( θ , θ ˙ ) θ ˙ + τ G + J T ( θ ) F e x t \tau =M\left( \theta \right) \ddot{\theta}+c\left( \theta ,\dot{\theta} \right) \dot{\theta}+\tau _G+J^{\mathrm{T}}\left( \theta \right) \mathcal{F} _{\mathrm{ext}} τ=M(θ)θ¨+c(θ,θ˙)θ˙+τG+JT(θ)Fext
F e x t \mathcal{F} _{\mathrm{ext}} Fext : applied from the body to environment
回顾:
J i J_{\mathrm{i}} Ji : body/link i Jacobian , V i ∣ 6 × 1 = J i ∣ 6 × n θ ˙ ∣ n × 1 \left. \mathcal{V} _{\mathrm{i}} \right|_{6\times 1}=\left. J_{\mathrm{i}} \right|_{6\times \mathrm{n}}\left. \dot{\theta} \right|_{\mathrm{n}\times 1} Vi∣6×1=Ji∣6×nθ˙ n×1
τ ∈ R n \tau \in \mathbb{R} ^n τ∈Rn , τ \tau τ play two major roles :
- generate motion
- generate force/torque
3.2 Properties of Dynamics Model of Multi-Body Systems
Only cpnsider body 2’s effect

4. Forward Dynamics Algorithms
4.1 Forward Dynamics Problem
τ = M ( θ ) θ ¨ + c ( θ , θ ˙ ) θ ˙ + τ G + J T ( θ ) F e x t = M ( θ ) θ ¨ + h ( θ , θ ˙ ) \tau =M\left( \theta \right) \ddot{\theta}+c\left( \theta ,\dot{\theta} \right) \dot{\theta}+\tau _G+J^{\mathrm{T}}\left( \theta \right) \mathcal{F} _{\mathrm{ext}}=M\left( \theta \right) \ddot{\theta}+h\left( \theta ,\dot{\theta} \right) τ=M(θ)θ¨+c(θ,θ˙)θ˙+τG+JT(θ)Fext=M(θ)θ¨+h(θ,θ˙)
-
Inverse dynamics : τ ← R N E A ( θ , θ ˙ , θ ¨ , F e x t ) \tau \gets RNEA\left( \theta ,\dot{\theta},\ddot{\theta},\mathcal{F} _{\mathrm{ext}} \right) τ←RNEA(θ,θ˙,θ¨,Fext) —— O ( N ) O\left( N \right) O(N) complexity
RNEA can work directly with a givenURDF-United Robotics Description Formatmodel (kinematic tree + joint model + dynamic parameters). It does not require explicit formula for M ( θ ) , h ( θ , θ ˙ ) M\left( \theta \right),h\left( \theta ,\dot{\theta} \right) M(θ),h(θ,θ˙) -
Forward dynamics : Given ( θ , θ ˙ ) , τ , F e x t \left( \theta ,\dot{\theta} \right) ,\tau ,\mathcal{F} _{\mathrm{ext}} (θ,θ˙),τ,Fext , find θ ¨ \ddot{\theta} θ¨
Calculate h ( θ , θ ˙ ) = c ( θ , θ ˙ ) θ ˙ + τ G + J T F e x t h\left( \theta ,\dot{\theta} \right) =c\left( \theta ,\dot{\theta} \right) \dot{\theta}+\tau _{\mathrm{G}}+J^{\mathrm{T}}\mathcal{F} _{\mathrm{ext}} h(θ,θ˙)=c(θ,θ˙)θ˙+τG+JTFext
Caculate mass matrix M ( θ ) = ∑ i = 1 N J i T I i J i M\left( \theta \right) =\sum_{i=1}^N{{J_{\mathrm{i}}}^{\mathrm{T}}\mathcal{I} _{\mathrm{i}}J_{\mathrm{i}}} M(θ)=∑i=1NJiTIiJi
Solve M θ ¨ = τ − h ⇒ θ ¨ = M − 1 ( τ − h ) M\ddot{\theta}=\tau -h\Rightarrow \ddot{\theta}=M^{-1}\left( \tau -h \right) Mθ¨=τ−h⇒θ¨=M−1(τ−h): This is not the most efficient way to find θ ¨ \ddot{\theta} θ¨
4.2 Caculations of h and M
Denote our inverse dynamics algorithm : τ = R N E A ( θ , θ ˙ , θ ¨ , F e x t ) = M θ ¨ + h \tau =RNEA\left( \theta ,\dot{\theta},\ddot{\theta},\mathcal{F} _{\mathrm{ext}} \right) =M\ddot{\theta}+h τ=RNEA(θ,θ˙,θ¨,Fext)=Mθ¨+h
-
Calculation of h h h : obviously , τ = h \tau =h τ=h if θ ¨ = 0 \ddot{\theta}=0 θ¨=0. Therefore, h h h can be computed via : h ( θ , θ ˙ ) = R N E A ( θ , θ ˙ , 0 , F e x t ) h\left( \theta ,\dot{\theta} \right) =RNEA\left( \theta ,\dot{\theta},0,\mathcal{F} _{\mathrm{ext}} \right) h(θ,θ˙)=RNEA(θ,θ˙,0,Fext)
-
Calculation of M M M : Note h ( θ , θ ˙ ) = c ( θ , θ ˙ ) θ ˙ + τ G + J T F e x t h\left( \theta ,\dot{\theta} \right) =c\left( \theta ,\dot{\theta} \right) \dot{\theta}+\tau _{\mathrm{G}}+J^{\mathrm{T}}\mathcal{F} _{\mathrm{ext}} h(θ,θ˙)=c(θ,θ˙)θ˙+τG+JTFext
Set G = 0 , F e x t = 0 , θ ˙ = 0 G=0,\mathcal{F} _{\mathrm{ext}}=0,\dot{\theta}=0 G=0,Fext=0,θ˙=0 , then h ( θ , θ ˙ ) = 0 h\left( \theta ,\dot{\theta} \right) =0 h(θ,θ˙)=0 , ⇒ τ = M ( θ ) θ ¨ \Rightarrow \tau =M\left( \theta \right) \ddot{\theta} ⇒τ=M(θ)θ¨
We can compute the j j jth colum of M ( θ ) M\left( \theta \right) M(θ) by calling the inverse algorithm
τ = M : , j ( θ ) = R N E A ( 0 , 0 , θ ¨ 0 , j , 0 ) \tau =M_{:,\mathrm{j}}\left( \theta \right) =RNEA\left( 0,0,\ddot{\theta}_{0,\mathrm{j}},0 \right) τ=M:,j(θ)=RNEA(0,0,θ¨0,j,0) , θ ¨ 0 , j \ddot{\theta}_{0,\mathrm{j}} θ¨0,j is a vector with all zeros except for a 1 at the j j jth entry
θ ¨ 0 , 1 = [ 1 0 ⋮ 0 ] , τ = [ M 1 ( θ ) ⋯ M n ( θ ) ] , M 1 , j ( θ ) = τ θ ¨ 0 , 1 \ddot{\theta}_{0,1}=\left[ \begin{array}{c} 1\\ 0\\ \vdots\\ 0\\ \end{array} \right] ,\tau =\left[ \begin{matrix} M_1\left( \theta \right)& \cdots& M_{\mathrm{n}}\left( \theta \right)\\ \end{matrix} \right] ,M_{1,\mathrm{j}}\left( \theta \right) =\tau \ddot{\theta}_{0,1} θ¨0,1= 10⋮0 ,τ=[M1(θ)⋯Mn(θ)],M1,j(θ)=τθ¨0,1 -
A more effiicient algorithm for computing M M M is the
Composite-Rigid-Body Algorithm(CRBA). Details can be found in Featherstone’s Book
4.3 Forward Dynamics Algorithms
Now assume we have θ , θ ˙ , τ , M ( θ ) , h \theta ,\dot{\theta},\tau ,M\left( \theta \right) ,h θ,θ˙,τ,M(θ),h , then we can immediately compute θ ¨ \ddot{\theta} θ¨ as θ ¨ = M − 1 ( τ − h ) \ddot{\theta}=M^{-1}\left( \tau -h \right) θ¨=M−1(τ−h) —— θ ¨ = F D ( τ , θ , θ ˙ , F e x t ) \ddot{\theta}=FD\left( \tau ,\theta ,\dot{\theta},\mathcal{F} _{\mathrm{ext}} \right) θ¨=FD(τ,θ,θ˙,Fext)
两种看似不同的求解思路,之间的关系是等同的
This provides a 2nd-order differential equation in R n \mathbb{R} ^n Rn , we can easily simulate the joint trajectory over any time period (under given ICs θ 0 , θ ˙ 0 \theta ^0,\dot{\theta}^0 θ0,θ˙0)
Computational Complexity:
RNEA : O ( N ) O\left( N \right) O(N)
h ( θ , θ ˙ ) = R N E A ( θ , θ ˙ , 0 , F e x t ) h\left( \theta ,\dot{\theta} \right) =RNEA\left( \theta ,\dot{\theta},0,\mathcal{F} _{\mathrm{ext}} \right) h(θ,θ˙)=RNEA(θ,θ˙,0,Fext) : O ( N ) O\left( N \right) O(N)
M ( θ ) M\left( \theta \right) M(θ) : O ( N 2 ) O\left( N^2 \right) O(N2)
M − 1 ( θ ) M^{-1}\left( \theta \right) M−1(θ) : O ( N 3 ) O\left( N^3 \right) O(N3)
Most efficient forward dynamics algorithm :
Articulate-Body Algorithm(ABA): O ( N ) O\left( N \right) O(N)
4.4 More Discussions
M ( θ ) M\left( \theta \right) M(θ) : Mass matrix , M T ( θ ) = M ( θ ) M^{\mathrm{T}}\left( \theta \right) =M\left( \theta \right) MT(θ)=M(θ), also positive semi-definite.
M ( θ ) = ∑ i = 1 N J i T I i J i M\left( \theta \right) =\sum_{i=1}^N{{J_{\mathrm{i}}}^{\mathrm{T}}\mathcal{I} _{\mathrm{i}}J_{\mathrm{i}}} M(θ)=∑i=1NJiTIiJi—— I i \mathcal{I} _{\mathrm{i}} Ii Inertia matrix symmetric / postive semi-definite
There are many equivalent ways to define c ( θ , θ ˙ ) c\left( \theta ,\dot{\theta} \right) c(θ,θ˙) , they are all related to the same product c ( θ , θ ˙ ) θ ˙ c\left( \theta ,\dot{\theta} \right) \dot{\theta} c(θ,θ˙)θ˙ —— common and confusing

Typical expression for c c c : c i j = ∑ k = 1 1 2 ( ∂ M i j ∂ θ k + ∂ M i k ∂ θ j − ∂ M j k ∂ θ i ) θ ˙ k , Γ i j k = 1 2 ( ∂ M i j ∂ θ k + ∂ M i k ∂ θ j − ∂ M j k ∂ θ i ) c_{\mathrm{ij}}=\sum_{k=1}^{}{\frac{1}{2}\left( \frac{\partial M_{\mathrm{ij}}}{\partial \theta _{\mathrm{k}}}+\frac{\partial M_{\mathrm{ik}}}{\partial \theta _{\mathrm{j}}}-\frac{\partial M_{\mathrm{jk}}}{\partial \theta _{\mathrm{i}}} \right)}\dot{\theta}_{\mathrm{k}},\varGamma _{\mathrm{ijk}}=\frac{1}{2}\left( \frac{\partial M_{\mathrm{ij}}}{\partial \theta _{\mathrm{k}}}+\frac{\partial M_{\mathrm{ik}}}{\partial \theta _{\mathrm{j}}}-\frac{\partial M_{\mathrm{jk}}}{\partial \theta _{\mathrm{i}}} \right) cij=∑k=121(∂θk∂Mij+∂θj∂Mik−∂θi∂Mjk)θ˙k,Γijk=21(∂θk∂Mij+∂θj∂Mik−∂θi∂Mjk) chrostoffel symbol
c ( θ , θ ˙ ) c\left( \theta ,\dot{\theta} \right) c(θ,θ˙) defind using Γ i j k \varGamma _{\mathrm{ijk}} Γijk satisies : [ M ˙ − 2 c ] \left[ \dot{M}-2c \right] [M˙−2c] skew symmetric matrix ( n × n n\times n n×n)
M ( θ ) , c ( θ , θ ˙ ) , τ G M\left( \theta \right) ,c\left( \theta ,\dot{\theta} \right) ,\tau _G M(θ),c(θ,θ˙),τG all depend on I i \mathcal{I} _{\mathrm{i}} Ii linearly
相关文章:

[足式机器人]Part4 南科大高等机器人控制课 Ch09 Dynamics of Open Chains
本文仅供学习使用 本文参考: B站:CLEAR_LAB 笔者带更新-运动学 课程主讲教师: Prof. Wei Zhang 南科大高等机器人控制课 Ch09 Dynamics of Open Chains 1. Introduction1.1 From Single Rigid Body to Open Chains1.2 Preview of Open-Chain …...

概率论复习
第一章:随机概率及其概率 A和B相容就是 AB 空集 全概率公式与贝叶斯公式: 伯努利求概率: 第二章:一维随机变量及其分布: 离散型随机变量求分布律: 利用常规离散性分布求概率: 连续性随机变量…...
ES客户端RestHighLevelClient的使用
1 RestHighLevelClient介绍 默认情况下,ElasticSearch使用两个端口来监听外部TCP流量。 9200端口:用于所有通过HTTP协议进行的API调用。包括搜索、聚合、监控、以及其他任何使用HTTP协议的请求。所有的客户端库都会使用该端口与ElasticSearch进行交互。…...

GitHub入门命令介绍
GitHub是当今最受欢迎的代码托管平台之一,它提供了强大的版本控制和协作功能。 对于初学者来说,熟悉GitHub的基本命令非常重要。下面介绍一些常用的GitHub命令。 一、安装Git 1. Windows系统:在Windows上使用GitHub之前,您需要先…...
EasyExcel 简单导入
前边写过使用easyexcel进行简单、多sheet页的导出。今天周日利用空闲写一下对应简单的导入。 重点:springboot、easyExcel、桥接模式; 说明:本次使用实体类student:属性看前边章节内容; 1、公共导入service public …...

Termux搭建nodejs环境
安装nodejs ~ $ pkg install nodejs使用http-server搭建文件下载服务 先安 http-server 并启动 # 安装 http-server 包 ~ $ npm install -g http-server# 启动 http-server 服务 ~ $ http-server Starting up http-server, serving ./http-server version: 14.1.1http-serve…...
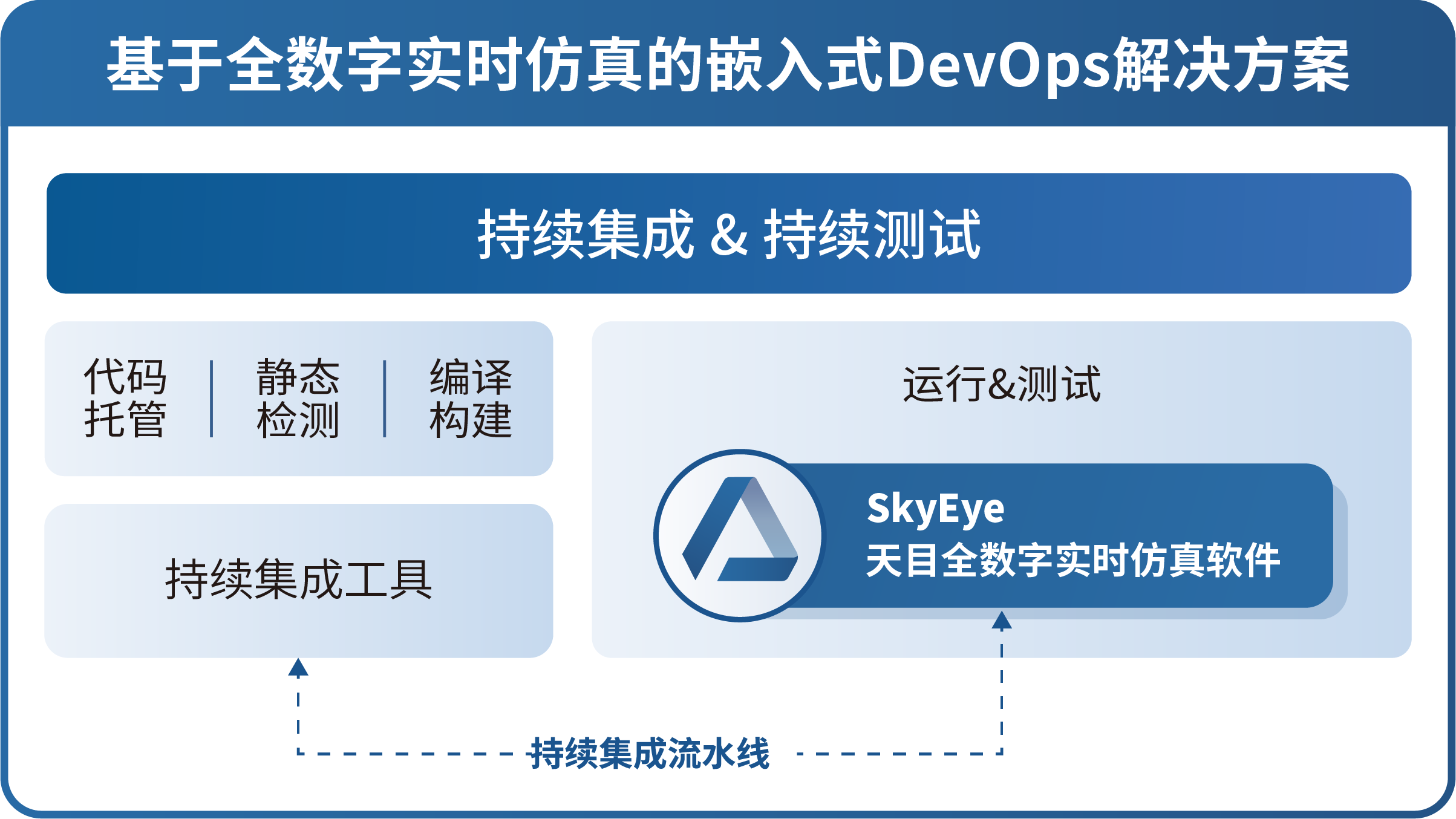
喜报丨迪捷软件入选2023年浙江省信息技术应用创新典型案例
12月6日,浙江省经信厅公示了2023年浙江省信息技术应用创新典型案例入围名单。本次案例征集活动,由浙江省经信厅、省密码管理局、工业和信息化部网络安全产业发展中心联合组织开展,共遴选出24个优秀典型解决方案,迪捷软件“基于全数…...
)
C语言连接zookeeper客户端(不能完全参考官网教程)
准备过程 1.通过VStudio 远程连接linux的开发环境; 2.g环境,通过MingW安装; 3.必须要安装好pthread.h的环境,不管是windows端(linux 可视化端开发就不管这个)还是linux端; 4.需要准备zookeeper…...

python排序
0. 背景 Python排序功能十分强大,可以进行基本排序或自定义排序。Python中提供两种不同的排序方法对各种各样的数据类型进行排序。 1. 使用sorted()函数排序 排序主要是对相同数据类型的元素进行的,包括数值和字符串两种数据类型。 1.1 对数值进行排…...
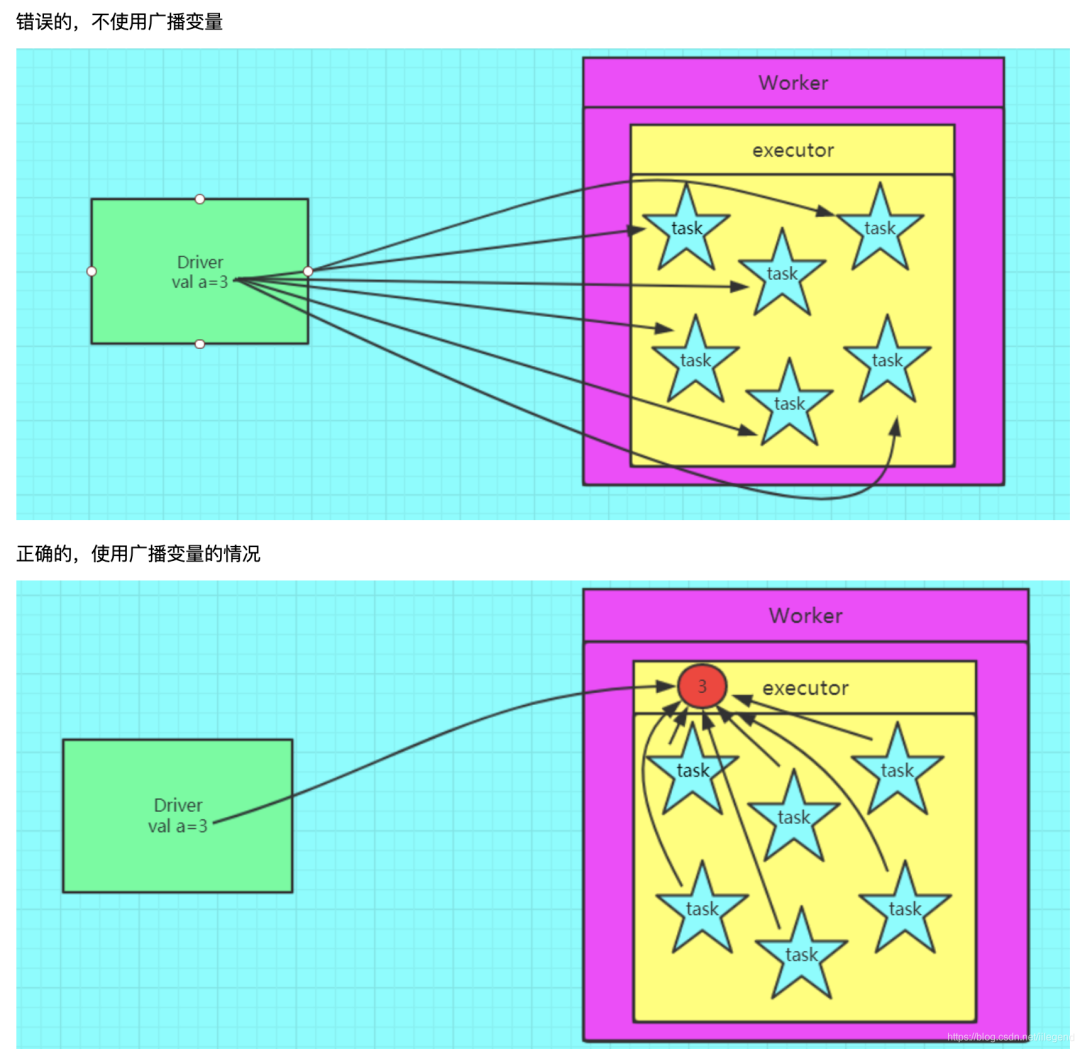
【Spark精讲】Spark Shuffle详解
目录 Shuffle概述 Shuffle执行流程 总体流程 中间文件 ShuffledRDD生成 Stage划分 Task划分 Map端写入(Shuffle Write) Reduce端读取(Shuffle Read) Spark Shuffle演变 SortShuffleManager运行机制 普通运行机制 bypass 运行机制 Tungsten Sort Shuffle 运行机制…...
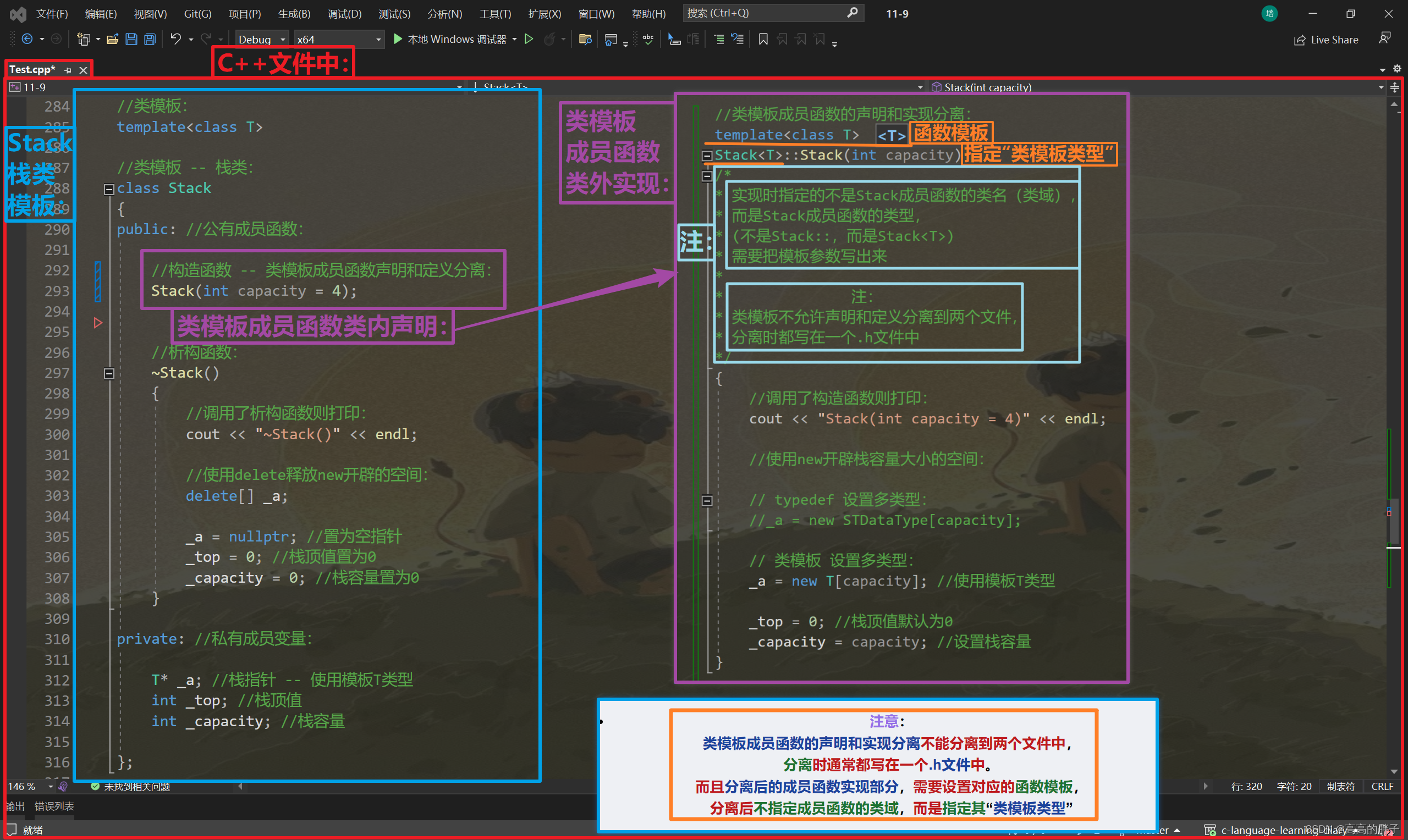
【C++初阶】八、初识模板(泛型编程、函数模板、类模板)
相关代码gitee自取: C语言学习日记: 加油努力 (gitee.com) 接上期: 【C初阶】七、内存管理 (C/C内存分布、C内存管理方式、operator new / delete 函数、定位new表达式) -CSDN博客 目录 一 . 泛型编程 二 . 函数模板 函数模板…...

珠海数字孪生赋能工业智能制造,助力制造业企业数字化转型
珠海数字孪生赋能工业智能制造,助力制造业企业数字化转型。数字孪生是利用物理模型、传感器更新及运行历史数据,集成多物理量、多尺度的仿真过程。巨蟹数科数字孪生通过构建物理车间与虚拟车间之间的有效映射并实时反馈机制,实现物理车间与虚…...
HarmonyOS开发实战:如何实现一个运动排名榜页面
HarmonyOS开发实战:如何实现一个运动排名榜页面 代码仓库: 运动排名榜页面 项目介绍 本项目使用声明式语法和组件化基础知识,搭建一个可刷新的排行榜页面。在排行榜页面中,使用循环渲染控制语法来实现列表数据渲染,…...

2019年第八届数学建模国际赛小美赛D题安全选举的答案是什么解题全过程文档及程序
2019年第八届数学建模国际赛小美赛 D题 安全选举的答案是什么 原题再现: 随着美国进入一场关键性的选举,在确保投票系统的完整性方面进展甚微。2016年总统大选期间,唐纳德特朗普因被指控受到外国干涉而入主白宫,这一问题再次成为…...

vivado 创建实施约束
创建实施约束 在您有了一个合成的网表之后,您可以将它与XDC文件一起加载到内存中,或者Tcl脚本已启用以进行实现。当加载XDC以便验证和更正任何不能应用的约束。在某些情况下,合成网表中的对象名称与精心设计。如果是这种情况,则必…...

【代码分析】MPI
代码解读 问题 model/AdaMPI.py:21 为什么下降分辨率model.CPN.unet.FeatMaskNetwork 为什么用的是mask,unet? MPI class MPIPredictor(nn.Module):def __init__(self,width384,height256,num_planes64,):super(MPIPredictor, self).__init__()self.…...
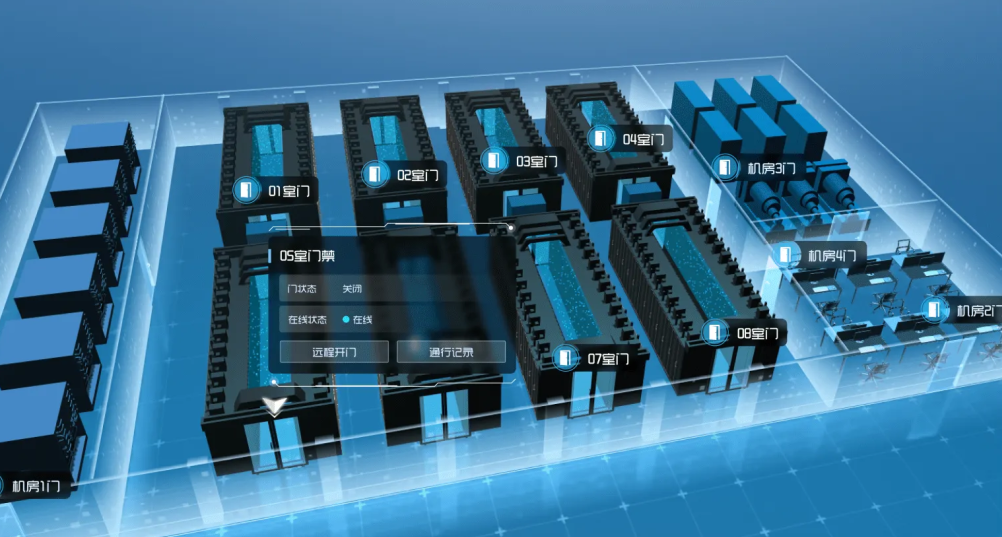
数字孪生Web3D智慧机房可视化运维云平台建设方案
前言 进入信息化时代,数字经济发展如火如荼,数据中心作为全行业数智化转型的智慧基座,重要性日益凸显。与此同时,随着东数西算工程落地和新型算力网络体系构建,数据中心建设规模和业务总量不断增长,机房管理…...

飞天使-docker知识点12-docker-compose
文章目录 docker-compose命令启动单个容器重启容器停止和启动容器停止和启动所有容器演示一个简单示范 docker-compose 部署有依赖问题 Docker Compose 是一个用于定义和运行多容器 Docker 应用程序的工具。它允许您使用简单的 YAML 文件来配置应用程序的服务、网络和存储等方…...
快速排序(一)
目录 快速排序(hoare版本) 初级实现 问题改进 中级实现 时空复杂度 高级实现 三数取中 快速排序(hoare版本) 历史背景:快速排序是Hoare于1962年提出的一种基于二叉树思想的交换排序方法 基本思想:…...

GO的sql注入盲注脚本
之间学习了go的语法 这里就开始go的爬虫 与其说是爬虫 其实就是网站的访问如何实现 因为之前想通过go写sql注入盲注脚本 发现不是那么简单 这里开始研究一下 首先是请求网站 这里貌似很简单 package mainimport ("fmt""net/http" )func main() {res, …...
51c自动驾驶~合集58
我自己的原文哦~ https://blog.51cto.com/whaosoft/13967107 #CCA-Attention 全局池化局部保留,CCA-Attention为LLM长文本建模带来突破性进展 琶洲实验室、华南理工大学联合推出关键上下文感知注意力机制(CCA-Attention),…...

汽车生产虚拟实训中的技能提升与生产优化
在制造业蓬勃发展的大背景下,虚拟教学实训宛如一颗璀璨的新星,正发挥着不可或缺且日益凸显的关键作用,源源不断地为企业的稳健前行与创新发展注入磅礴强大的动力。就以汽车制造企业这一极具代表性的行业主体为例,汽车生产线上各类…...

在四层代理中还原真实客户端ngx_stream_realip_module
一、模块原理与价值 PROXY Protocol 回溯 第三方负载均衡(如 HAProxy、AWS NLB、阿里 SLB)发起上游连接时,将真实客户端 IP/Port 写入 PROXY Protocol v1/v2 头。Stream 层接收到头部后,ngx_stream_realip_module 从中提取原始信息…...

鸿蒙中用HarmonyOS SDK应用服务 HarmonyOS5开发一个医院查看报告小程序
一、开发环境准备 工具安装: 下载安装DevEco Studio 4.0(支持HarmonyOS 5)配置HarmonyOS SDK 5.0确保Node.js版本≥14 项目初始化: ohpm init harmony/hospital-report-app 二、核心功能模块实现 1. 报告列表…...
Mac软件卸载指南,简单易懂!
刚和Adobe分手,它却总在Library里给你写"回忆录"?卸载的Final Cut Pro像电子幽灵般阴魂不散?总是会有残留文件,别慌!这份Mac软件卸载指南,将用最硬核的方式教你"数字分手术"࿰…...
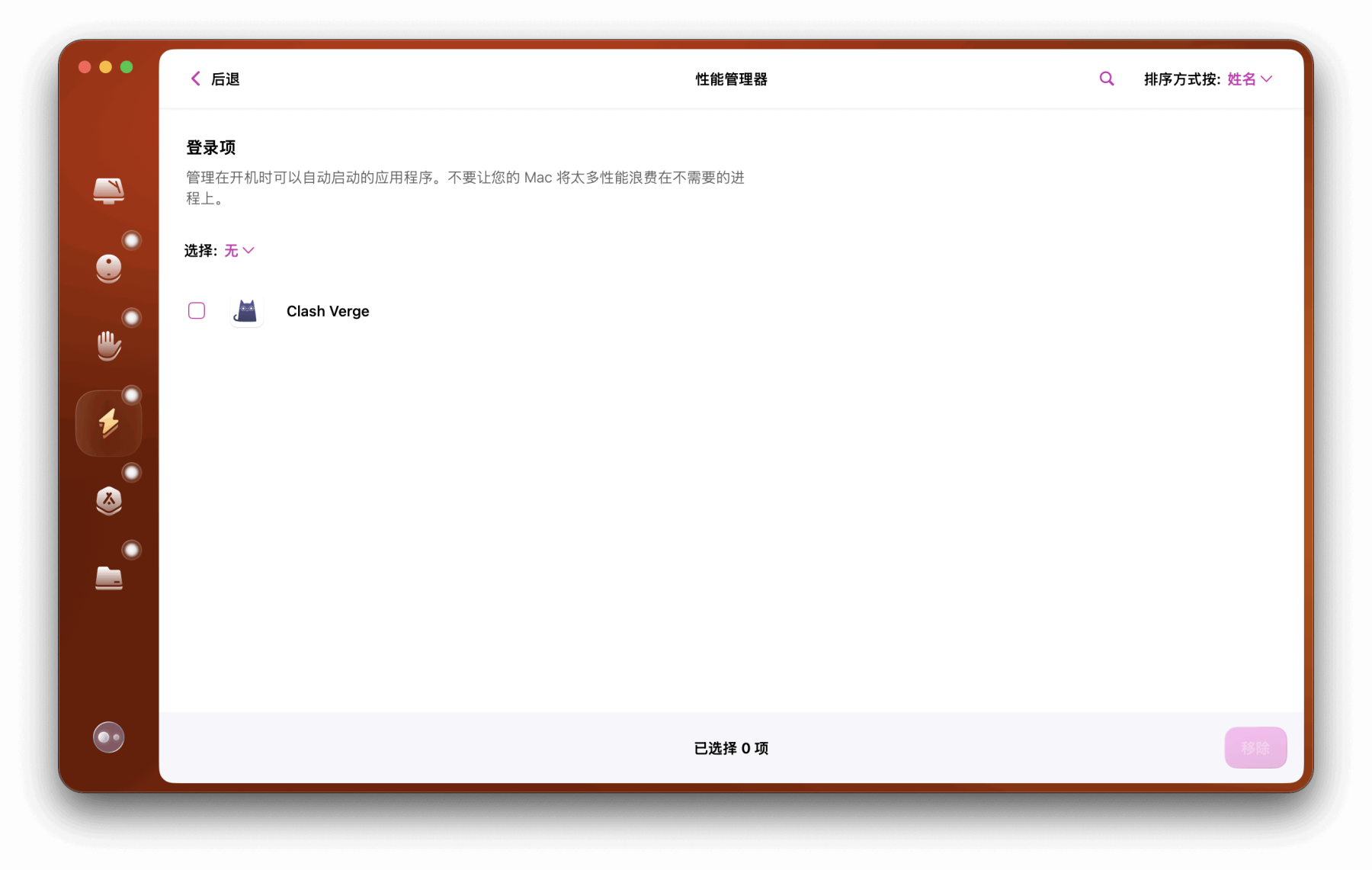
令牌桶 滑动窗口->限流 分布式信号量->限并发的原理 lua脚本分析介绍
文章目录 前言限流限制并发的实际理解限流令牌桶代码实现结果分析令牌桶lua的模拟实现原理总结: 滑动窗口代码实现结果分析lua脚本原理解析 限并发分布式信号量代码实现结果分析lua脚本实现原理 双注解去实现限流 并发结果分析: 实际业务去理解体会统一注…...

CMake控制VS2022项目文件分组
我们可以通过 CMake 控制源文件的组织结构,使它们在 VS 解决方案资源管理器中以“组”(Filter)的形式进行分类展示。 🎯 目标 通过 CMake 脚本将 .cpp、.h 等源文件分组显示在 Visual Studio 2022 的解决方案资源管理器中。 ✅ 支持的方法汇总(共4种) 方法描述是否推荐…...
关键领域软件测试的突围之路:如何破解安全与效率的平衡难题
在数字化浪潮席卷全球的今天,软件系统已成为国家关键领域的核心战斗力。不同于普通商业软件,这些承载着国家安全使命的软件系统面临着前所未有的质量挑战——如何在确保绝对安全的前提下,实现高效测试与快速迭代?这一命题正考验着…...
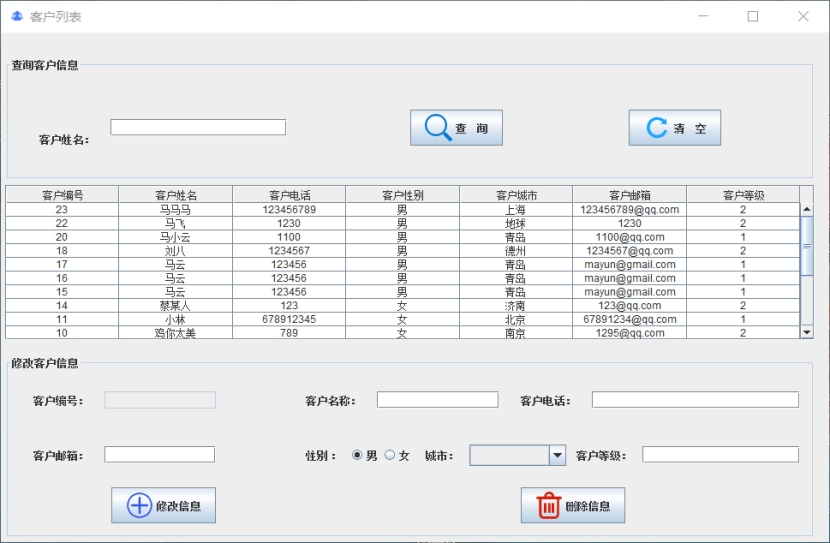
基于Java+MySQL实现(GUI)客户管理系统
客户资料管理系统的设计与实现 第一章 需求分析 1.1 需求总体介绍 本项目为了方便维护客户信息为了方便维护客户信息,对客户进行统一管理,可以把所有客户信息录入系统,进行维护和统计功能。可通过文件的方式保存相关录入数据,对…...

Bean 作用域有哪些?如何答出技术深度?
导语: Spring 面试绕不开 Bean 的作用域问题,这是面试官考察候选人对 Spring 框架理解深度的常见方式。本文将围绕“Spring 中的 Bean 作用域”展开,结合典型面试题及实战场景,帮你厘清重点,打破模板式回答,…...
